Received: July, 2011
Fluorine Notes, 2011, 78, 7-8
Forecasting Glass Transition Temperatures of Fluorocontaining Polymers
A. N. Kollar, V. A. Gubanov
Federal State Unitary Enterprise S.Y. Lebedev Research Institute for synthetic rubber (FSUE "NIISK")
e-mail: vniisk@vniisk.cplus.ru
Abstract: The analysis of Tc (Tg, Glass Transition Temperature) dependence on both length and structure of the chain connecting triazine rings in perfluorinated polymers synthesized by the authors made it possible to improve the algorithm for Tc calculation developed earlier by A.A.Askadskii and G.L.Slonimsky. The proposed variant of Tc calculation allows reliable forecasting of cold resistance behaviour of both carbochain- and heterochain-fluoropolymers and tailoring of promising materials with the best properties.
Keywords:fluoropolymers, structure - glass transition temperature, calculation of glass transition temperature, tailoring of advanced structures.
The synthesis of polymers with pre-designed structures is among of the modern chemistry and physics challenges. The Glass Transition Temperature (Tc) is one of the most significant polymer properties. Tcforecasting prior to the polymer synthesis, and reasoning from the chemical structure of its repeating units seems to be a topical problem. A considerable number of studies have already been devoted to the problems of Tc relation to the polymer chemical structure. In general, those calculations based on the assumption that the macromolecule repeating unit may be divided into a number of atomic groups with additive contributions to Tcindependent of the environment. Its mathematical expression is as follows:
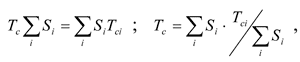
Here Tci is characteristic contribution of a group into Tc; and Si is the mass coefficient assigned to the group.
In the most of polymers Tcis estimable with accuracy +20 K. The methods for the Tc calculation based on such assumption was considered in [1-5] along with some other methods. However, the main disadvantages of the techniques offered by those authors are their non-flexibility and low accuracy. Another method different from those mentioned was introduced by A.A.Askadskii and G.L.Slonimsky for the calculation of Tcin amorphous polymers [6]. Basing on the temperature dependence of polymer packing factor they derived an equation that relates the polymer Tcto some parameters of the chemical structure of a macromolecule repeating unit:

Here 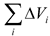 is the characteristic mole
volume of the repeating unit made up of the volumes of atoms that enter into the composition of the
repeating unit (Van der Waals volume);
is the characteristic mole
volume of the repeating unit made up of the volumes of atoms that enter into the composition of the
repeating unit (Van der Waals volume);
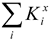 - is an additive value (with volume
dimensionality) related to the packing factor and to the coefficient of volumetric expansion of the
polymer body (efficient volume of the chain repeating unit);
- is an additive value (with volume
dimensionality) related to the packing factor and to the coefficient of volumetric expansion of the
polymer body (efficient volume of the chain repeating unit);
NА is Avogadro constant equal to 6.023·10 23;
A is a parameter of any linear
polymer equal to 1.435.
The computation 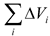 is based on the assumptions
suggested in [7]. Here
is based on the assumptions
suggested in [7]. Here 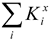 is a
sum of values Kxi that characterize each element and every type of
intermolecular interactions. The solution of a redundant equation system based on (1) resulted in
Kxi values shown in table 1.
is a
sum of values Kxi that characterize each element and every type of
intermolecular interactions. The solution of a redundant equation system based on (1) resulted in
Kxi values shown in table 1.
Table 1. Numerical values for Kxi [6].
# |
Element or type of intermolecular interaction |
Conventional sign |
Numerical value
|
|
1 2 3 4 5 6 7 8 |
Carbon Oxygen (>C=O) Hydrogen Nitrogen Chlorine Hydrogen bond Dipole-dipole interaction* Coefficient of symmetry** |
KXC KXO KXH KXN KXCl KXh KXd KXn |
10.739 3.925 –1.248 9.520 7.242 7.338 7.827 10.500 |
* – KXd introduced in vinyl polymers when hydrogen in its main or side-chain is substituted
with a radical of any type except phenyl.
** – KXn introduced in case that all aromatic
cores in the main-chain are substituted in n-position.
In further studies [8, 9] two more increments were added: main-chain oxygen KXOO equal to minus 5.244, and main-chain nitrogen KXNO that is 2.185.
In [10] the computation scheme was further improved to determine Tcin fluoropolymers (mainly, perfluorinated triazine polymers, PFT). To this end some novel Kxi were introduced, their numerical values were determined (Table 2) and Tc were calculated in a number of polymers.
Table 2. Numerical values for Kxi [10]
# |
Element or type of intermolecular interaction |
Symbol |
Numerical value
|
|
1 2 3 4 5 |
Fluorine Oxygen (in chain) Dipole-dipole interaction due to ≡CF group Dipole-dipole interaction due to –CF2– group ДDipole-dipole interaction due to –CF3 group |
KXF KXO KXd1 KXd2 KXd3 |
2.944 0.215 2.080 3.312 3.610 |
For homotypic PTF polymers with hexafluoroethylene oxide (HFPO) in their main or side chains the calculated Tc deviated from its experimental value less than by 2%.However, PFT polymers with tetrafluoroethylene oxide (TFEO) links in their main chains and most of synthesized PFT do not fit in the above calculation scheme.
In table 3 experimental Tc values for some polymers synthesized by us are compared to Tc calculated using the published data [10].
Table 3. Experimental and calculated Tc in polymers.
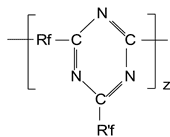
From table 3 one may conclude that the available calculation procedure does not allow Tcforecasting in novel polymer and hence requires updating. Recently Marchionni [12] developed an equation for non-branched perfluoropolyether homo- or co-polymers:
TC= 200–80(O/C).
The extrapolation of this equation resulted in Tc equal to 200 K (–73°C) for polytetrafluoroethylene (O/C=0), and in Tcequal to 120 K (–153°C) for homopolymer with structure –(CF2O)–. Below it will be shown that those values differ considerably from our computation results and point to the impossibility of diverging precise functions reasoning only from atomic ratios.
Furthermore A.A.Askadskii proposed a calculation scheme based on the assumption about voluminal dilatation of solid bodies [9,13]. At present such calculation schemes have been developed for quantitative assessment of virtually all polymer properties [14], and the possibility of computer modelling of the synthesis of polymers with designed characteristics has been proved. However, A.A.Askadskii was correct to mention that the applicability of the method decreases as the increments required for calculation accuracy grow in number; and the predictive force of the method may drop to zero, if a novel polymer requires a novel increment to be introduced. Therefore, some polymers e.g., fluoropolymers (never considered by A.A. Askadskii) do not fit in available computational schemes.
We synthesized a series of PFT polymers with different structures of their main and side chains. The analysis of Tc dependence on the length and structure of their triazine rings cross-linking has shown as follows:
1. The introduction of oxygen into PFT polymer side-chain decreases the impact of the main-chain oxygen on the polymer Tc; the said decrease is less obvious for main chains with tetrafluoroethylene oxide links.
2. For polymers with perfluorooxamethylene side-chains only the length of its main-chain influence on the polymer Tc notwithstanding the presence or absence of oxygen in HFPO links.
3. Starting with CF3O(CF2O)2CF2– radical in side-chain the pattern of Tc dependence on the length of main-chain changes, and Tc decreases with the length of polymer main-chain.
Therefore, the effect of oxygen in main-chain differs from that in side-chain, and oxygen in perfluorooxamethylene group inserted into the polymer side-chain provides the maximal effect.
Following [10], our calculation of Tcin fluoropolymers based on equation (1) with some new increments introduced into it.
Reasoning from the obtained experimental results and taking into account that the contribution of a group does not depend on the nature of adjacent groups only for ideal additivity, and that the best correlation between calculated and experimental results is achieved when group contributions are used instead of those atomic, we made the assumptions as follows:
1. Two KXO increment values must be used in the computational scheme: KXO main for oxygen in the polymer main-chain, and KXO side for oxygen in the polymer side-chain.
2. The minimal KXO value is that of oxygen in perfluorooxamethylene chain.
3. For oxygen surrounded by carbon-containing groups the increment is calculated as the mean of group effects proportional to the number of carbons –Cn–O–Cm– :
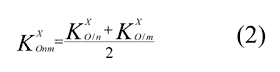
4. An additional increment KXO Tr must be introduced (for triazine ring).
Basing on those assumptions and starting from experimental Tc in synthesized polymers, and using numerical KXi values published in [6, 10], we solved the redundant equation system based on (1) and determined new KXi values presented in Table 4.
Table 4. Numerical values for KXi.
# |
Element |
Conventional sign |
Numerical value
|
| 1 | Oxygen in side oxamethylene chain | KXO side | –3.760 |
| 2 | Oxygen in HFPO links of main chain | KXO1 main | 1.324 |
| 3 | Oxygen in TFEO links of main chain | KXO2 main | –1.220 |
| 4 | Triazine ring | KX Tr | 32.077 |
Van der Waals volume for a chain link is calculated as a sum of volume increments ΔVi shown in Table 5 and taken from published data [10, 15].
Table 5. Increments for van der Waals atomic group volume.
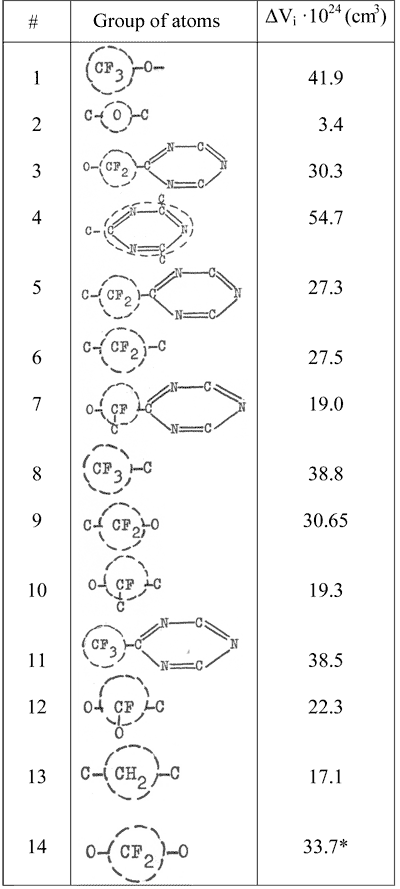
* Calculated by us
Those newly determined increments we used to calculate Tc in synthesized PTF polymers. A comparison between calculated and experimental values shows that both positive and negative deviations and their maximal values are virtually the same (mean square deviation was ±3.1 K), thus confirming the correctness and validity of the calculation scheme.
Figure 1 shows the calculated dependence of Tc on
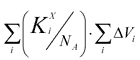 , that is essentially fits all experimental
data.
, that is essentially fits all experimental
data.
However, there are some points that poorly fit the calculated curve. They belong to polymers with long perfluoroalkyl side chains. Their deviations of experimental Tc from those calculated with the proposed technique were negative and the quadratic mean deviation was –12.5. For those structures the nature of increments must be revised. It is obvious that in those polymers the impact of their main-chain carbons on Tc differs from that of side-chain carbons.
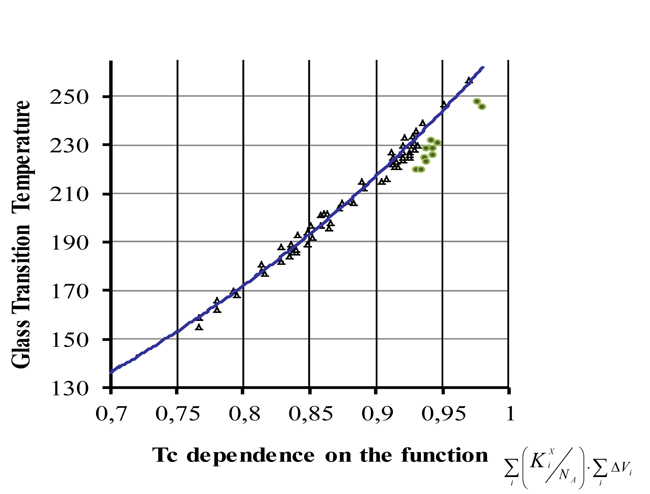
Fig. 1.
To check our earlier assumptions we calculated Tcin some perfluoropolyethers, and the results are present in Table 6.
Table 6.Calculated and experimental TC in perfluoropolyethers.
# |
Polyether |
TC (K)
|
TC (K)*
|
Reference |
| 1 | –[(CF2CF2O)p(CF2O)q]n–
p/q = 0.6¸0.7 |
142 | 136.5 | [16] |
| 2 | –(CF2CF2CF2CF2O)n– | 208 | 221 | [17] |
| 4 | –(CFCF2O)n– | CF3 |
201 | 204.5 | NIISK |
| 5 | –(CF2O)n– | - | 102.5 | – |
* Tc calculation in polymers with trifluoromethyl side-groups are carried out with KXO2 main increment, while in polymers without side-chains with KXO side.
From table 6 one may see that the convergency of experimental and calculated results is not very high, and its mean square deviation is ±7.7. However, taking into account that the method for Tc determination differed from that used by us, and that those polymers are mostly low-molecular, this deviation may be considered as satisfactory. Therefore, we estimated the limit Tcin polyethers, its calculated value in polydifluoromethylene oxide is –170.5°C.
The proposed method for the calculation of glass transition temperature was applied as well in the calculation of Tcof carbochain co-polymers of perfluoroalkylvinyl ethers and vinylidenfluoride [(CH2CF2)3CF(Rf)CF2]n manufactured in our institute by the emulsion polymerization. For them the deviation of calculated values from those determined experimentally was somewhat higher than for perfluorooxaalkyltetrazine: ±5.8 K.
Table 7. Structures, Tc, lg Tcand 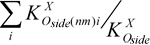 соpolymers of vinylidenfluoride and perfluorovinyl ethers –(CH2CF2CH2CF2CH2CF2CF(Rf)CF22)n–
соpolymers of vinylidenfluoride and perfluorovinyl ethers –(CH2CF2CH2CF2CH2CF2CF(Rf)CF22)n–

On the basis of table 7 were derived the dependence of lgTc on 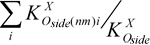 , expressed by the following equation:
, expressed by the following equation:
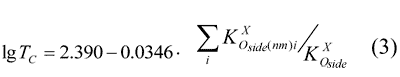
or in less complicated form:
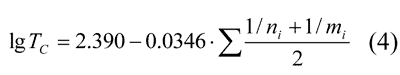
This dependence makes it possible to forecast Tc in polymers with the above-mentioned compositions with any perfluorovinyl ether. Similar equations may be derived for any comb-shaped polymers with perfluorooxaalkyl side-chains. E.g., for polymers –[(CF2CF(Rf)O)4CF2O]n–
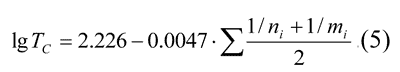
For polymers with general formula –[PN(OCH2Rf)2]n–
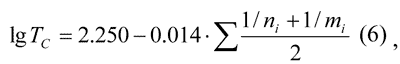
It fits very well experimental data (mean square deviation does not exceed ±2). The general equation for comb-shaped polymers with perfluorooxaalkyl side-chains is as follows:
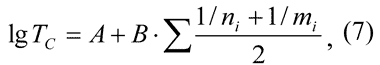
Here А and В are constants for this polymer series. Those equations allow sufficient accuracy in the forecasting of Tc in such polymers and rule out complicated calculations using the above algorithm.
As it was already mentioned we have studied experimentally the dependence of Tc in PTF polymers with perfluorooxamethylene side chains on the length of their main chain that contained oxygen in the form of HFPO links, or containing no oxygen. Those were straight-line functions with Tc decreasing with the main chain length. It was interesting to study similar functions for polymers with oxygens in TFEO included into their main chains. Being not able to synthesize such polymers currently we calculated their Tc using the refined calculation scheme. The results are shown in Table 8.
Table 8.Calculated TC in polymers.

# |
Rf |
TC (K) calculated |
| 1 2 3 4 5 6 7 8 |
–(CF2OCF2)2– –(CF2OCF2)3– –(CF2OCF2)4– –(CF2OCF2)5– –(CF2OCF2)6– –(CF2OCF2)7– –(CF2OCF2)8– –(CF2OCF2)9– |
139.5 143.5 147 149.5 152 154 156 157.5 |
From Table 8 one may see for the polymers with TFEO-oxygens the same inverse relationship between the main chain length and Tc, and as the main chain length increases Tc tend to a constant value. It should be noted that for those polymers their Tc is 15÷30K lower than those of similar polymers containing oxygens in OHFP-links of their main chains. It must be due to large contribution of TFEO-link oxygens into the total chain flexibility.
When going from HFPO-linked oxygen to TFEO-linked oxygen KXO main values change from positive to negative and approach KXO side of perfluorooxamethylene links, i.e. the main chain becomes less flexible and less different from the side-chain. Taking it into account and having in mind Tc calculated for perfluoropolyethers (Table 6) one may assume that in the case of perfluorooxamethylene main chain its KXO3 main is equal to KXO side for perfluorooxamethylene side-chain, i.e KXO3 main is –3.76. The difference between KXO2 main and KXO1 main, is equal to that between KXO3 main and KXO2 main , thus confirming to some extent our above assumptions. Reasoning from it we calculated Tc in polymers with perfluorooxamethylene links in their main and side-chains (table 9).
Table 9.Calculated TC in polymers.

# |
Rf |
TC (K) calculated |
| 1 2 3 4 5 6 7 |
–CF2OCF2OCF2– –CF2O(CF2O)2CF2– –CF2O(CF2O)3CF2– –CF2O(CF2O)4CF2– –CF2O(CF2O)5CF2– –CF2O(CF2O)6CF2– –CF2O(CF2O)7CF2– |
132 129.5 127.5 126 124.5 123 122 |
From table 9 one may see that in this case the dependence of Tc on the main chain length, i.e. as the main chain length increases Tc decreases and tends to a constant value. Obviously, the minimal Tcpossible in polymers with above mentioned structures must be about 123 K (–150°C).
We used thus developed calculation scheme to determine Tc in a number of oxygen-containing fluoropolymers, mostly not synthesized yet. Those polymers’ structures and Tc are shown in table 10.
Table 10. Calculated and experimental Tc in oxygen-containing fluoropolymers
From table 10 one may see that the drop of Tc in polymers with long perfluorooxamethylene side-chains is more prominent in polymers with rigid main chain (perfluorocarbons). Similar behaviour was observed in comb-shaped polymers with –CH2– links in their side-chains [1]. It should be mentioned as well that polymers with flexible main chains and long perfluorooxamethylene side-chains have nearly the same Tcthat and as the number of –CF2O– links increases their TC tends to Tc of polyperfluorooxamethylene, that is 102.5 K (–170.5°C). The same trend is valid for other polymers but evening-out of their Tc occurs at higher content of –CF2O– links. Therefore, one may suggest that for any comb-shaped polymers with perfluorooxamethylene links in their side-chains Tc is not less than 102.5K (–170.5°C) that is the minimal Tc value for fluoropolymers.
Examples of vitrification temperature calculation in fluoropolymers
Calculation of Tc in polymer structure as follows:
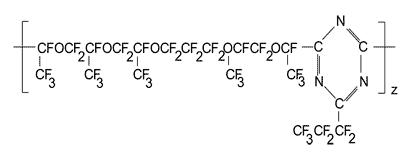
Van der Waals volume for the polymer link involves five atomic oxygen volumes plus five volumes of –CF2– groups linked to oxygen and aliphatic carbon; plus two volumes of–CF2– groups linked to two aliphatic carbons; plus three volumes of ≡CF groups linked to oxygen and two aliphatic carbons; plus six volumes of CF3 groups linked to aliphatic carbon; triazine ring; plus two volumes of ≡CF groups linked to oxygen, and to aliphatic and aromatic carbons; plus one volume of –CF2– group linked to aliphatic and aromatic carbons.
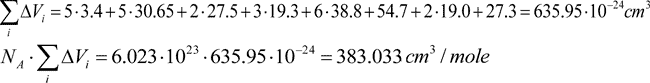
The effective volume of repeating link 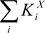 is a sum of corresponding KXi values.
is a sum of corresponding KXi values.
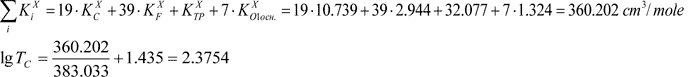
TC = 237.5 K
Calculation of Tc in polymer with the structure as follows:
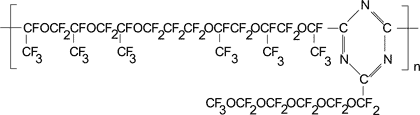
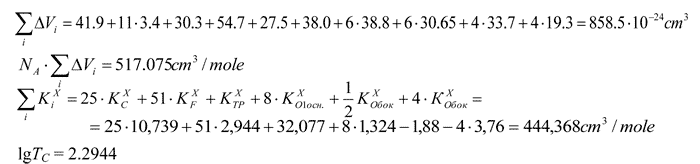
TC = 197 K
For oxygen related to triazine ring KXO is taken as ½KXO for oxygen surrounded of another adjacent group, if KXO<0 and 2KXO, if KXO>0.
Calculation of Tc in polymer with the structure as follows:
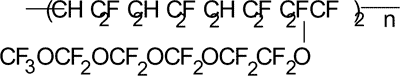

KXO for oxygen adjacent to the main chain is taken to be equal to ½ of KXO for oxygen surrounded of another adjacent group. For соpolymers with similar structures it is ½KXh.
Calculation of Tc in polymer with the structure as follows:
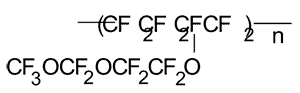
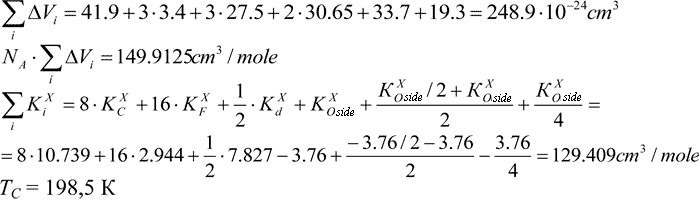
For those copolymers we take ½KXd.
Calculation of Tc in polymer with the structure as follows:
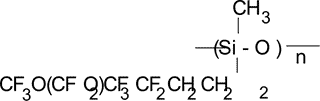
In order to calculate Tc in such polymers we calculated Vi for 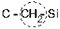 group 16.0·10–24 cm3. The numerical KXO value
for oxygen connected to two silicons KXOSi was calculated from the experimental
data on Tc in polymers that was equal to –7.02 cm3/mole.
group 16.0·10–24 cm3. The numerical KXO value
for oxygen connected to two silicons KXOSi was calculated from the experimental
data on Tc in polymers that was equal to –7.02 cm3/mole.
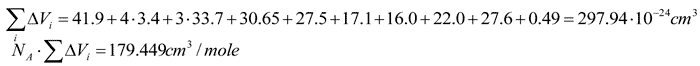
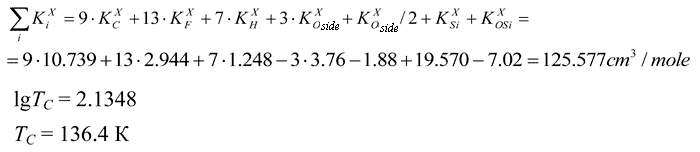
Therefore, basing on the investigation of the impact of ether bond located in the side- or main chain of PFT polymers on their Tc we have further developed the algorithm earlier proposed by Askadskii and Slonimsky for the computational forecasting of glass transitional temperatures in fluoropolymers and proved its applicability for a large series of polymer structures. Those calculations have shown the principal possibility of synthesis of triazine polymers with perfluorooxamethylene structures in all its fragments with Tc up to 123 K (–150°C) and the synthesis of polyperfluorooxamethylene with Tc equal to 102.5 K (–170.5°C). The minimal achievable Tc in any fluoropolymer is 102.5 K. An empiric equation is proposed for the forecasting of Tc in any comb-shaped polymers with perfluorooxaalkyl side-chains, and its applicability is shown.
The results obtained with the help of the developed calculation scheme made it possible to produce perfluorotriazine liquids with wide temperature range of applicability [18-21]. Those liquids are used for the base in plastic lubricants and antioxidant-anticorrosion additives to perfluoropolyethers and plastic lubricants based on them.
The study was supported financially by the Ministry of Education and Science of the Russian Federation, the State Contract # 02.523.12.3027.
References
2. Geoffrey Gee // Contemporary Physics. 1970. V. 11. N 3. P. 313-334
3. H.G. Weyland, P.J. Hoftyzer, D.W. Van Krevelen // Polymer. 1970. V. 11. N 2. P. 79-87
4. Van Krevelen D.V.“Svojstva i himicheskoe stroenie polimerov”. M.:Khimiya. 1976. 414c.
5. Becker Robert // Fazerforschung und Textiltechnic. Zeitschrift fürpolymerforschung, 1978. V. 29. N 6. P. 361-385
6. Askadskij A.A., Slonimskij G.L. // Vysokomol. soed. 1971. T. 13 A. No 8. S. 1917-1919
7. Kitajgorodskij A.I. “Organicheskaya kristallokhimiya”. M.: AN SSSR. 1955. S. 24
8. Askadskij A.A. // Uspekhi khimii. 1977. T. 46, vyp. 6. S. 1122-1151
9. Askadskij A.A. “Struktura i svojstva teplostojkih polimerov”. M.:Khimiya. 1981. S. 93
10. Yarosh A.A., Askadskij A.A., Krukovskij S.P.// Vysokomol. soed. 1974. T.16 A. No 3. S. 527-533
11. Bergshtejn L.A.“Laboratornyj praktikum po tekhnologii rezin”, L.: Khimiya. 1978. 224s.
12. Marchionni G., Ajroldi G. // Soc. Automot. Eng. 1992. sp-936, P. 87-96
13. Askadskij A.A., Matveev Yu.I.“Khimicheskoe stroenie i fizicheskiesvojstva polimerov”. M.: Khimiya. 1993. S.248
14. Askadskij A.A. // Vysokomol. soed. 1995. T. 37 B . No 2. S. 332-357
15. Slonimskij G.L., Askadskij A.A., Kitajgorodskij A.I. // Vysokomol. soed. 1970. T.12 A. No 3. S. 494-506
16. Marchionni G, Ajroldi G., Pezzin G. // Eur. Polym. J. 1988. V. 24. N 12. P. 1211-1216
17. Rice D.E. // J. Polym. Sci. Part B: Polym. Letters. 1968. V. 6. N 5. P.335
18. Patent US 5.681 921, 28.10.97.
19. Patent US 5.783 659, 21.07.98.
20. Patent US 5.789 532 4.08.98.
21. Patent US 5.942 598, 24.08.99.
Recommended for publication by Prof. V. Gubanov
Fluorine Notes, 2011, 78, 7-8
